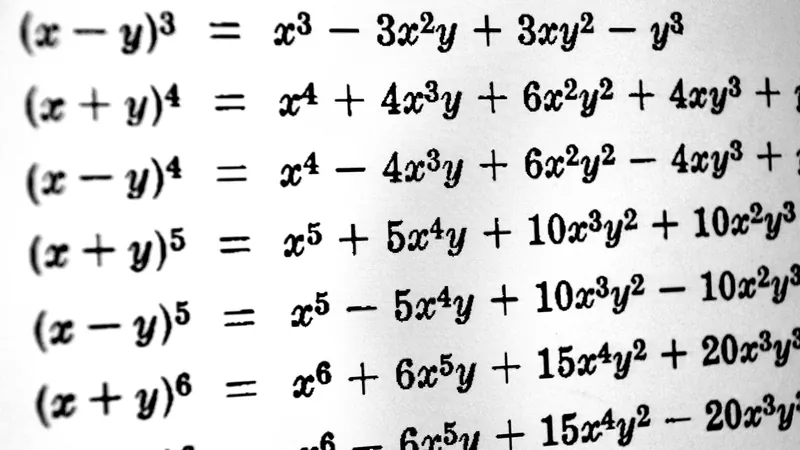
Revolutionary Breakthrough: Mathematicians Uncover New Method to Solve Complex Polynomial Equations
2025-05-02
Author: Arjun
Polynomial equations have always been the bedrock of modern science, influencing everything from celestial mechanics and computer graphics to market forecasts. While many high school students can breeze through basic polynomial equations, the enigmas posed by higher-order polynomials have long baffled even the most seasoned mathematicians.
However, a pair of brilliant minds from the University of New South Wales—mathematician Norman Wildberger and independent computer scientist Dean Rubine—have unveiled a groundbreaking method to tackle these challenging equations. Their revolutionary approach was published on April 8 in the esteemed journal, The American Mathematical Monthly.
But what exactly is a polynomial? Simply put, it’s an algebraic equation with variables raised to non-negative powers, such as x² + 5x + 6 = 0. This concept boasts roots that reach back to ancient civilizations like Egypt and Babylon.
Although mathematicians have mastered simple polynomials, those involving powers above four have proved to be a completely different beast. The traditional methods for two-, three-, and four-degree polynomials often rely on radicals—roots of exponential numbers that can represent irrational numbers, which lead to endless decimal expansions, much like the number pi.
Even though it’s possible to use these radicals to gain approximate solutions for specific higher-order polynomials, a universal formula that applies to all has remained elusive. As Wildberger succinctly put it, finding such a solution would require "an infinite amount of work and a hard drive larger than the universe."
In a daring twist, Wildberger and Rubine sidestepped the use of radicals altogether. They leaned into the power of polynomial extensions, specifically power series—an infinitely long string of terms that harness the powers of x, commonly employed in solving geometric challenges. This intriguing method draws from a branch of mathematics known as combinatorics.
At the core of their approach lies the fascinating Catalan numbers, a sequence associated with the ways to decompose a polygon into triangles. First introduced by Mongolian mathematician Mingantu around 1730 and later discovered by Leonhard Euler in 1751, these numbers became the key to unlocking higher-order polynomial solutions. Wildberger and Rubine dubbed their innovative extension "the Geode."
The implications of the Geode are monumental, with vast potential applications in fields like computer science and graphics. As Wildberger boldly stated, "This is a dramatic revision of a basic chapter in algebra." Prepare for a shift in mathematical understanding!


 Brasil (PT)
Brasil (PT)
 Canada (EN)
Canada (EN)
 Chile (ES)
Chile (ES)
 Česko (CS)
Česko (CS)
 대한민국 (KO)
대한민국 (KO)
 España (ES)
España (ES)
 France (FR)
France (FR)
 Hong Kong (EN)
Hong Kong (EN)
 Italia (IT)
Italia (IT)
 日本 (JA)
日本 (JA)
 Magyarország (HU)
Magyarország (HU)
 Norge (NO)
Norge (NO)
 Polska (PL)
Polska (PL)
 Schweiz (DE)
Schweiz (DE)
 Singapore (EN)
Singapore (EN)
 Sverige (SV)
Sverige (SV)
 Suomi (FI)
Suomi (FI)
 Türkiye (TR)
Türkiye (TR)
 الإمارات العربية المتحدة (AR)
الإمارات العربية المتحدة (AR)